CBSE Class 10 Maths Chapter 2 Polynomials Ex 2.1 || NCERT Solutions for Class 10 Maths Chapter 2: Polynomials (English Medium)
Explore the NCERT Solutions for Class 10 Maths Chapter 2: Polynomials (English Medium), with detailed explanations for Exercise 2.1. This resource is designed to simplify complex polynomial concepts, helping Class 10 Maths students grasp the subject effortlessly. For any queries regarding NCERT Solutions for Class 10 Maths Chapter 2 Polynomials Ex 2.1, feel free to leave a comment, and we’ll respond as soon as possible.

CBSE Class 10 maths chapter 2 Ex 2.1 || NCERT Solutions for Class 10
Maths Chapter 2
Exercise-2.1
CBSE Class 10 maths chapter 2 Ex 2.1 || NCERT Solutions for Class 10 Maths Chapter 2 Exercise 2.1
Download the Math Ninja App NowThe number of zeroes for any graph is the number of values of x for which y
is equal to zero. And y is equal to zero at the point where a graph cuts x axis.
So, to find the number of zeroes of a polynomial, watch the number of times it cuts the x axis.
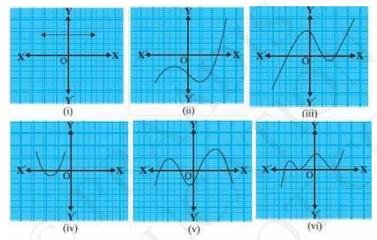
(i) The number of zeroes is 0 as the graph does not cut the \( x \)-axis at any point.
(ii) The number of zeroes is 1 as the graph intersects the x -axis at only 1 point.
(iii) The number of zeroes is 3 as the graph intersects the \( x \)-axis at 3 points.
(iv) The number of zeroes is 2 as the graph intersects the \( x \)-axis at 2 points.
(v) The number of zeroes is 4 as the graph intersects the \( x \)-axis at 4 points.
(vi) The number of zeroes is 3 as the graph intersects the \( x \)-axis at 3 points.
So, to find the number of zeroes of a polynomial, watch the number of times it cuts the x axis.
(i) The number of zeroes is 0 as the graph does not cut the \( x \)-axis at any point.
(ii) The number of zeroes is 1 as the graph intersects the x -axis at only 1 point.
(iii) The number of zeroes is 3 as the graph intersects the \( x \)-axis at 3 points.
(iv) The number of zeroes is 2 as the graph intersects the \( x \)-axis at 2 points.
(v) The number of zeroes is 4 as the graph intersects the \( x \)-axis at 4 points.
(vi) The number of zeroes is 3 as the graph intersects the \( x \)-axis at 3 points.