NCERT Solutions for Class 10 Maths Chapter 3: Pair of Linear Equations in Two Variables || CBSE Class 10 Maths Chapter 3 Pair of Linear Equations in Two Variables solutions Ex 3.1 Math Solution

Given: Seven years ago, Aftab was seven times as old as his daughter and after 3 years Aftab will be 3 times as old as his daughter.
To Represent the situations algebraically and graphically we need to find the linear equations for these situations. Let present age of Aftab \( =\mathrm{x} \)
Let present age of his daughter \( =\mathrm{y} \)
Then, seven years ago the age of Aftab and his daughter must have been seven less than their present ages, Age of Aftab seven years ago \( =\mathrm{x}- \) 7
Age of Daughter seven years ago \( =\mathrm{y}-7 \)
According to the question,
Seven years ago, Aftab was seven times as old as his daughter, So
\(x-7=7(y-7)\)
\(\Rightarrow x-7=7 y-49\)
\(\Rightarrow x=7 y-42\)
Now for finding different points of this equation, we can either take different values of x and put them in the equation to obtain values of y or vice versa
Putting \( y=5,6 \) and \(7\) in equation \( (i) \), we get,
\(\text { For } y=5\)
\(x=7 \times 5-42\)
\(=35-42=-7 \text { For } x=6\)
\(x=7 \times 6-42\)
\(=42-42=0 \text { For } y=7\)
\(x=7 \times 7-42\)
\(=49-42=7\)
\(\begin{array}{|l|l|l|l|}
\hline x & -7 & 0 & 7 \\
\hline y & 5 & 6 & 7 \\
\hline
\end{array}\)
Thus we got 3 points to plot on graph for this equation.
Three years from now,
Age of Aftab \( =x+3 \)
Age of Daughter \( =y+3 \)
According to the question,
\(\Rightarrow x+3=3(y+3) \Rightarrow x+3=3 y+9 \Rightarrow x=3 y+6\)
Now for finding different points of this equation, we can either take different values of \( x \) and put them in equation to obtain values of \( y \) or vice versa
Putting \( \mathrm{x}=0,3 \) and \(6\)
\(\begin{array}{|l|l|l|l|}
\hline x & 0 & 3 & 6 \\
\hline y & -2 & -1 & 0 \\
\hline
\end{array}\)
Thus we got 3 points to plot on graph for this equation.
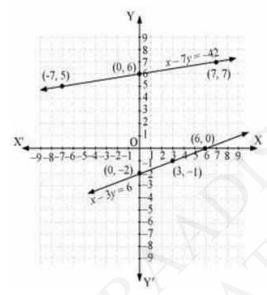
Algebraic representation
\(x-7 y=-42\) (i)
\(x-3 y=6\) (ii)
Graphical representation:

We need to form linear equations for the situations.
Let cost of one bat \( = \) Rs. x
Let cost of one ball = Rs. y
In first case, three bats and 6 balls cost him 3900 rupees. Therefore our equation becomes
\(\Rightarrow 3 x+6 y=3900\)...(i)
Dividing equation by 3 both side
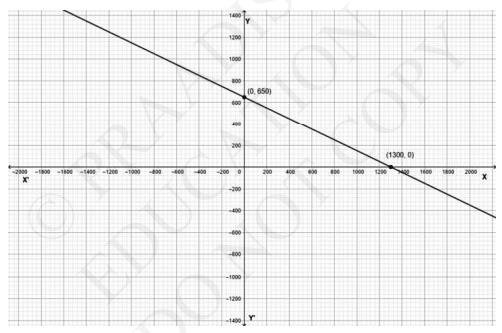
\(\Rightarrow x+2 y=1300\)
\(\Rightarrow x=1300-2 y\)
For plotting the equation of graph, take different values of \( y \) and obtain the value of \( x \) from equation or you can do vice versa. At \( y=0 \Rightarrow x= \) \( 1300-2(0) \Rightarrow \mathrm{x}=1300 \) Now finding the value at \( \mathrm{x}=0 \Rightarrow 0=1300 \) \( 2 \mathrm{y} \Rightarrow 2 \mathrm{y}=1300 \Rightarrow \mathrm{y}=650 \)
\(\begin{array}{|l|l|l|}
\hline X & 0 & 1300 \\\hline Y & 650 & 0 \\\hline
\end{array}\)
From above points, we make the graph as follows [Graphic representation]

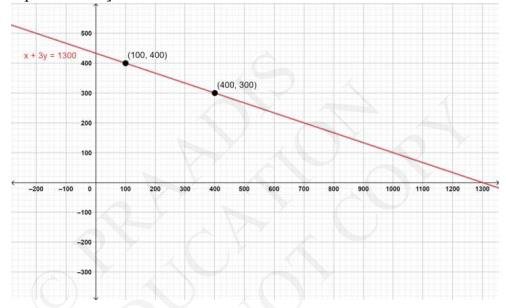
In second case he buys one bat and 3 balls for 1300 , therefore,
\(\Rightarrow x+3 y=1300\)...(ii) \(\quad \) [Algebraic representation]
\( \Rightarrow x=1300-3 y\)
\(\text { At } y=400 \)
\(\Rightarrow \mathrm{x}=1300-3(400) \)
\(\Rightarrow \mathrm{x}=100 \text { At } \mathrm{y}=300 \)
\(\Rightarrow \mathrm{x}=1300-3(300) \)
\(\Rightarrow \mathrm{x}=400\)
\(\begin{array}{|l|l|l|}
\hline x & 100 & 400 \\
\hline y & 400 & 300 \\
\hline
\end{array}\)
From above points, we make the graph as follows, [Graphical representation]
s

Let the cost of each kg of apples = Rs. X
Let the cost of each kg of grapes \( = \) Rs. Y
According to the question,
Cost of 2 kg of Apples \( =2 \mathrm{x} \)
Cost of 1 kg of grapes \( =\mathrm{y} \)
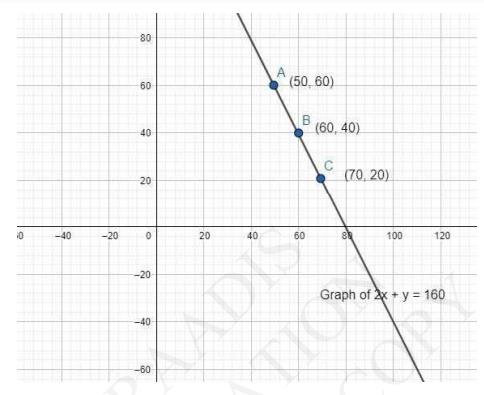
\(2 x+y=160\ldots(i)\)
\(2 x=160-y\)
\(x=\frac{16-y}{2}\)
Putting \( y=20,40 \) and 60 we get,
\(x=\frac{(160-20)}{2}=70\)
\(x=\frac{(160-40))}{2}=60\)
\(x=\frac{(160-60)}{2}=50\)
\(\begin{array}{|l|l|l|l|}\hline X & 50 & 60 & 70 \\\hline Y & 60 & 40 & 20 \\\hline\end{array}\)
Algebraic Representation: \( 2 \mathrm{x}+\mathrm{y}=160 \)
Graphical Representation:

Now taking another case
Cost of 4 kg of apples and 2 kg of grapes is Rs.300.................................(Given)
So, \( 4 \mathrm{x}+2 \mathrm{y}=300 \ldots(ii)\)
Dividing the equation by 2 , we get,
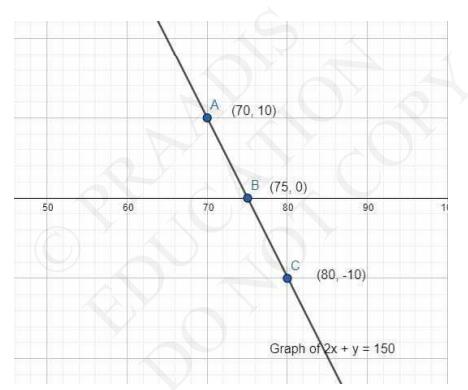
\(2 x+y=150\)
\(y=150-2 x\)
Putting \( \mathrm{x}=70,75 \) and 80 we get,
\(y=150-2 \times 70=10\)
\(y=150-2(75)=0\)
\(y=150-2(80)=150-160=-10\)
\(\begin{array}{|l|l|l|l|}
\hline X & 70 & 75 & 80 \\\hline Y & 10 & 0 & -10 \\
\hline\end{array}\)
Algebraic representation:
\(4 x+2 y=300\)
Graphical representation: