NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Get the complete NCERT Solutions for Class 10 Maths Chapter 12 : Areas Related to Circles, covering Exercise 12.2. This free resource helps you understand key concepts and solve problems with ease, perfect for CBSE Class 10 students preparing for exams using NCERT Maths materials. We hope the NCERT Solutions for Class 10 Maths Chapter 12 Areas Related to Circles Exercise 12.2 help you. If you have any queries regarding NCERT Maths Solutions Chapter 12 Areas Related to Circles Exercise 12.2, drop a comment below, and we will get back to you at the earliest.

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2
Exercise 12.2

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

1. Find the area of a sector of a circle with radius 6 cm if the angle of the sector is

$60^{\circ}$ .

Answer

Let

$\mathrm{OACB}$ be a sector of the circle making

$60^{\circ}$ angle at centre

$\mathrm{O}$ of the circle

Area of sector of angle

$\theta=\frac{\theta}{360} \times \pi r^{2}$
Area of sector

$\mathrm{OACB}=\frac{60}{360} \times \frac{22}{7} \times 6 \times 6$

$=\frac{132}{7} \mathrm{~cm}^{2}$
Hence,
The area of the sector of the circle making

$60^{\circ}$ at the centre of the circle is

$\frac{132}{7} \mathrm{~cm}^{2}$ .

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

2. Find the area of a quadrant of a circle whose circumference is 22 cm .

Answer

To find: Area of the quadrant of the circle
Given: Circumference of the circle
Let the radius of the circle be

$r$
Circumference

$=22 \mathrm{~cm}$
Circumference

$=2 \pi r$
Therefore,

$2 \pi r=22$

$r=\frac{22}{2 \pi}$

$=\frac{11}{\pi}$

Quadrant of circle means

$\frac{ 1 }{ 4 }$ th of the circle.
Area of such quadrant of the circle

$=\frac{1}{4} \pi r^{2}$

$=\frac{1}{4} \pi\left(\frac{11}{\pi}\right)^{2}$

$\frac{121}{\pi}$

$=\frac{121 \times 7}{4 \times 22}$

$=\frac{77}{8} \mathrm{~cm}^{2}$
Hence, The area of the quadrant of the circle is

$\frac{77}{8} \mathrm{~cm}^{2}$

3. The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes.

Answer

So 15 minutes

$=90^{\circ} 1 \min =\frac{90^{\circ}}{15}$

$=6^{\circ}$

$5 \mathrm{~min}=5 \times 6^{\circ}=30^{\circ}$ So 5 minutes subtend an angle of

$30^{\circ}$ .
Therefore, the area swept by the minute hand in 5 minutes will be the area of a sector of

$30^{\circ}$ in a circle of 14 cm radius.
Area swept by min hand

$=$ Area of sector

$=\frac{\theta}{360^{\circ}} \times \pi r^{2}$

$=\frac{30^{\circ}}{360^{\circ}} \times \frac{22}{7} \times(14)^{2}$

$=\frac{30^{\circ}}{360^{\circ}} \times \frac{22}{7} \times 14 \times 14$

$=\frac{154}{3} \mathrm{~cm}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

4. A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding:
(i) Minor segment (ii) Major sector (Use

$\pi=3.14$ )

Answer

Let

$\mathrm{AB}$ be the chord of the circle subtending

$90^{\circ}$ angle at centre

$\mathrm{O}$ of the circle.

$\text { Area of sector } \mathrm{OACB}=\frac{90^{\circ}}{360^{\circ}} \times 3.14 \times 10 \times 10$

$=\frac{90^{\circ}}{360^{\circ}} \times 3.14 \times 10 \times 10$

$=\frac{1}{4} \times 3.14 \times 10^{5} \times 10^{5}$

$=3.14 \times 25=78.5 \mathrm{~cm}^{2}$

$\mathrm{OAB}$ is a right angles triangle, Area of triangle

$\mathrm{OAB}=\frac{ 1 }{ 2 }$ (base

$\times$ height)
Area of

$\triangle \mathrm{OAB}=\frac{1}{2} \times \mathrm{OA} \times \mathrm{OB}$

$=\frac{1}{2} \times 10 \times 10$

$=50 \mathrm{~cm}^{2}$
Area of minor segment

$\mathrm{ACB}=$ Area of minor sector

$\mathrm{OACB}-$ Area of

$\triangle \mathrm{OAB}$

$=78.5-50$

$=28.5 \mathrm{~cm}^{2}$
ii) Let

$\mathrm{AB}$ be the chord of the circle subtending

$90^{\circ}$ angle at centre

$\mathrm{O}$ of the circle.
Area of major sector

$\mathrm{OACB}=$

$=\left(\frac{360^{\circ}-90^{\circ}}{360^{\circ}}\right) \times \pi r^{2}$

$=\left(\frac{270^{\circ}}{360^{\circ}}\right) \times \pi r^{2}$

$=\frac{ 3 }{ 4 } \times 3.14 \times 10 \times 10$

$=235.5 \mathrm{~cm}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

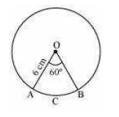
5. In a circle of radius 21 cm, an arc subtends an angle of

$60^{\circ}$ at the centre. Find:
(i) The length of the arc
(ii) Area of the sector formed by the arc
(iii) Area of the segment formed by the corresponding chord.

Answer

Radius

$(r)$ of circle

$=21 \mathrm{~cm}$
The angle subtended by the given arc

$=60^{\circ}$
Length of an arc of a sector of angle

$\theta=\frac{\theta}{360^{\circ}} \times 2 \pi r$
(i) Length of arc

$\mathrm{ACB}=\frac{60^{\circ}}{360^{\circ}} \times 2 \times \frac{22}{7} \times 21$

$=\frac{1}{6} \times 2 \times 22 \times 3$

$=22 \mathrm{~cm}$
(ii) Area of sector

$\mathrm{OACB}=\frac{\theta}{360^{\circ}} \pi r^{2}$

$=\frac{60^{\circ}}{360^{\circ}} \times \frac{22}{7} \times 21 \times 21$

$=\frac{1}{6} \times \frac{22}{7} \times 21 \times 21$

$=231 \mathrm{~cm}^{2}$
(iii) In

$\triangle \mathrm{OAB}$ ,
As

$\mathrm{OA}=\mathrm{OB}$

$\Rightarrow \angle \mathrm{OAB}=\angle \mathrm{OBA} \text { (Angles opposite to equal sides are equal) }$

$\Rightarrow \angle \mathrm{OAB}+\angle \mathrm{AOB}+\angle \mathrm{OBA}=180^{\circ}$

$\Rightarrow 2 \angle \mathrm{OAB}+60^{\circ}=180^{\circ}$

$\Rightarrow 2 \angle \mathrm{OAB}=180^{\circ}-60^{\circ}$

$\Rightarrow \angle \mathrm{OAB}=60^{\circ}$
Hence,

$\triangle \mathrm{OAB}$ is an equilateral triangle
Area of equilateral triangle

$=\frac{\sqrt{3}}{4}(S i d e)^{2}$

$\Rightarrow$ Area of

$\triangle \mathrm{OAB}=\frac{\sqrt{3}}{4}( Side)^{2}$

$\frac{\sqrt{3}}{4} \times(21)^{2}$

$=\frac{441 \sqrt{3}}{4} \mathrm{~cm}^{2}$
Area of segment

$\mathrm{ACB}=$ Area of sector

$\mathrm{OACB}$ - Area of

$\triangle \mathrm{OAB}$

$=\left(231-\frac{441 \sqrt{3}}{4}\right) \mathrm{cm}^{2}$

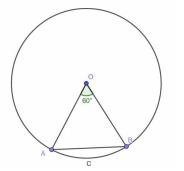
6. A chord of a circle of radius 15 cm subtends an angle of

$60^{\circ}$ at the centre. Find the areas of the corresponding minor and major segments of the circle.
(Use

$\pi=3.14$ and

$\sqrt{3}=1.73$ )

Answer

Radius

$(r)$ of circle

$=15 \mathrm{~cm}$
Area of sector

$\mathrm{OPRQ}=(\frac{ 60 }{ 360 }) \times \pi r^{2}$

$=\frac{ 1 }{ 6 } \times 3.14 \times(15)^{2}$

$=117.75 \mathrm{~cm}^{2}$
In

$\triangle \mathrm{OPQ}$ ,

$\angle \mathrm{OPQ}=\angle \mathrm{OQP}$ (As

$\mathrm{OP}=\mathrm{OQ})$

$\angle \mathrm{OPQ}+\angle \mathrm{OQP}+\angle \mathrm{POQ}=180^{\circ}$

$2 \angle \mathrm{OPQ}=120^{\circ}$

$\angle \mathrm{OPQ}=60^{\circ}$

$\Delta \mathrm{OPQ}$ is an equilateral triangle.

$\text { Area of } \Delta \mathrm{OPQ}=\frac{\sqrt{3}}{4} \times(Side)^{2}$

$=\frac{\sqrt{3}}{4} \times(15)^{2}$

$=\frac{225 \sqrt{3}}{4}$

$=56.25 \times \sqrt{3}$

$=97.3125 \mathrm{~cm}^{2}$
Area of segment

$\mathrm{PRQ}=$ Area of sector

$\mathrm{OPRQ}$ - Area of

$\triangle \mathrm{OPQ}$

$=117.75-97.3125$

$=20.4375 \mathrm{~cm}^{2}$
Area of major segment

$\mathrm{PSQ}$ = Area of circle - Area of segment

$\mathrm{PRQ}$

$=\pi(15)^{2}-20.4375$

$=3.14 \times 225-20.4375$

$=706.5-20.4375$

$=686.0625 \mathrm{~cm}^{2}$

7. A chord of a circle of radius 12 cm subtends an angle of

$120^{\circ}$ at the centre. Find the area of the corresponding segment of the circle. (Use

$\pi=3.14$ and

${\sqrt{3}} =1.73$ )

Answer

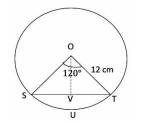
Let us draw a perpendicular

$\mathrm{OV}$ on chord

$\mathrm{ST}$ . It will bisect the chord

$\mathrm{ST}$ and the angle

$\mathrm{O}$ .

$\mathrm{SV}=\mathrm{VT}$
In

$\triangle \mathrm{OVS}$ ,

$\text { As, } \cos \theta=\frac{\text { Base }}{\text { Hypotenuse }}$

$\frac{O V}{O S}=\cos 60^{\circ}$

$\frac{O V}{12}=1$

$\mathrm{OV}=6 \mathrm{~cm}$

$\frac{s v}{s o}=\sin 60^{\circ}$

$\frac{s v}{12}=\frac{\sqrt{3}}{2}$

$\mathrm{SV}=6 {\sqrt{3}} \mathrm{~cm}$

$\mathrm{ST}=2 \times \mathrm{SV}$

$=2 \times 6{\sqrt{3}}$

$=12 {\sqrt{3}} \mathrm{~cm}$
Area of

$\Delta \mathrm{OST}=\frac{1}{2} \times 12 {\sqrt{3}} \times 6$

$=36 {\sqrt{3}}$

$=36 \times 1.73$

$=62.28 \mathrm{~cm}^{2}$
Area of sector

$\mathrm{OUST} =\frac{120}{360} \times \pi \times(12)^{2}$

$=\frac{1}{3} \times 3.14 \times 144$

$=150.72 \mathrm{~cm}^{2}$
Area of segment

$\mathrm{SUT} =$ Area of sector

$\mathrm{OUST}$ - Area of

$\triangle \mathrm{OST}$

$=150.72-62.28$

$=88.44 \mathrm{~cm}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

$Mathninja.in$

8. A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see Fig. 12.11). Find

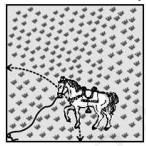
(i) The area of that part of the field in which the horse can graze
(ii) The increase in the grazing area if the rope were 10 m long instead of 5 m. (Use

$\pi=3.14$ )

Answer

From the figure, it can be observed that the horse can graze a sector of

$90^{\circ}$ in a circle of 5 m radius
(i) Area that can be grazed by horse

$=$ Area of sector

$=\frac{90}{360} \times \pi r^{2}$

$=\frac{1}{4} \times 3.14 \times 5 \times 5$

$=19.625 \mathrm{~m}^{2}$

$=\frac{1}{4} \times 3.14 \times 5 \times 5$

$=19.625 \mathrm{~m}^{2}$
The area that can be grazed by the horse when the length of rope is 10

$\mathrm{m} \text { long }=\frac{90}{360} \times \pi(10)^{2}$

$=\frac{1}{4} \times 3.14 \times 100$

$=78.5 \mathrm{~m}^{2}$
(ii) Increase in grazing area

$=$ Area grazed by a horse now - Area grazed previously
( 78.5 - 19.625)

$=58.875 \mathrm{~m}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

9. A brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire is also used in making 5 diameters which divide the circle into 10 equal sectors as shown in Fig. 12.12. Find:
(i) The total length of the silver wire required
(ii) The area of each sector of the brooch

Answer

(i) To Calculate total length of wire required to make brooch, we need to find Circumference of Brooch and the length of 5 diameters of Brooch.
Diameter of Circle

$=35 \mathrm{~mm}\ Radius =\frac{\text { Diameter }}{2}$
Radius of Circle

$=\frac{ 35 }{ 2 } \mathrm{~mm}$ Circumference of Circle

$=2 \pi \mathrm{r}$
Circumference of circle

$=2 \times \frac{22}{7} \times \frac{35}{2}$
Circumference of circle

$=110 \mathrm{~mm}$
Total Length of wire required

$=$ Circumference of Circle + 5 x Diameter of Circle Total Length of wire required

$=110+5 \times 35$ Total Length of wire required

$=285 \mathrm{~mm}$
(ii) A complete Circle subtends an angle of

$360^{\circ} 10$ sectors

$=360^{\circ} 1$ sector will subtend

$=36^{\circ}$ .
Area of sector of Circle

$=\frac{\theta}{360^{\circ}} \pi r^{2}$
Area of Each Sector of Brooch

$=\frac{36^{\circ}}{360^{\circ}} \times \frac{22}{7} \times \frac{35}{2} \times \frac{35}{2}$
Area of Each sector

$=\frac{385}{4} \mathrm{~mm}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

10. An umbrella has 8 ribs which are equally spaced (see Fig.
12.13). Assuming umbrella to be a flat circle of radius 45 cm, find the area between the two consecutive ribs of the umbrella.

Answer

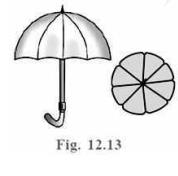
There are 8 ribs in the given umbrella. The area between two consecutive ribs is subtending

$\frac{360^{\circ}}{8}=45^{\circ}$ at the centre of the assumed flat circle
Now we know that area of a sector of a circle subtending an angle

$x$ is given by
Area

$=\frac{x}{360^{\circ}} \pi r^{2}$
Now as the value of

$x$ for given umbrella is

$45^{\circ}$
Area between two consecutive ribs

$=\frac{45^{\circ}}{360^{\circ}} \times \frac{22}{7} \times 45 \times 45$
Area between two consecutive ribs

$=\frac{11 \times 2025}{28}$
Area between two consecutive ribs

$=\frac{22275}{28} \mathrm{~cm}^{2}$

11. A car has two wipers which do not overlap. Each wiper has a blade of length 25 cm sweeping through an angle of

$115^{\circ}$ . Find the total area cleaned at each sweep of the blades.

Answer

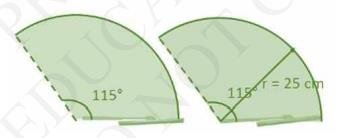
It can be seen from the figure that each blade of wiper would sweep an area of a sector of

$115^{\circ}$ in a circle with 25 cm radius

$\text { Area of sector }=\frac{\theta}{360^{\circ}} \pi r^{2}$

$=\frac{115^{\circ}}{360^{\circ}} \pi(25)^{2}$

$=\frac{115^{\circ}}{360^{\circ}} \times \frac{22}{7} \times 25 \times 25$

$=\frac{1581250}{2520} \mathrm{~cm}^{2}$
Area swept by 2 blades

$=2 \times \frac{1581250}{2520} \mathrm{~cm}^{2}$

$=\frac{1581250}{2520} \mathrm{~cm}^{2}$

$=1254.96 \mathrm{~cm}^{2}$

$=1255 \mathrm{~cm}^{2}(\text { approx })$

12. To warn ships for underwater rocks, a lighthouse spreads a red colored light over a sector of angle

$80^{\circ}$ to a distance of 16.5 km. Find the area of the sea over which the ships are warned. (Use

$\pi=3.14$ ).

Answer

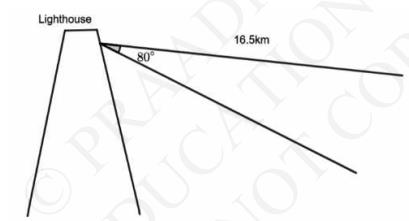
It can be seen from the figure that the lighthouse spreads light across a sector of

$80^{\circ}$ in a circle of 16.5 km radius
Area of sector

$\mathrm{OACB}=\frac{80}{360} \times \pi r^{2}$

$=\frac{2}{9} \times 3.14 \times 16.5 \times 16.5$

$=189.97 \mathrm{~km}^{2}$

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now

13. A round table cover has six equal design as shown in Fig.12.14. If the radius of the cover is 28 cm, find the cost of making the designs at the rate of Rs 0.35 per

$\mathrm{cm}^{2} .(\mathrm{Use}=\sqrt{3}=1.7)$

Answer

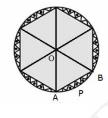
It can be concluded that these designs are segments of the circle.
Let us take segment APB.
Chord AB is a side of the regular hexagon.
And,
Each chord will substitute

$\frac{360}{6}=60^{\circ}$ at the centre of the circle
In

$\triangle \mathrm{OAB}$ ,

$\angle \mathrm{OAB}=\angle \mathrm{OBA}$ ( As

$\mathrm{OA}=\mathrm{OB})$

$\angle \mathrm{AOB}=60^{\circ}$

$\angle \mathrm{OAB}+\angle \mathrm{OBA}+\angle \mathrm{AOB}=180^{\circ}$

$2 \angle \mathrm{OAB}=180^{\circ}-60^{\circ}$

$=120^{\circ}$

$\angle \mathrm{OAB}=60^{\circ}$
Hence,

$\Delta \mathrm{OAB}$ is an equilateral triangle.
Area of

$\triangle \mathrm{OAB}=\frac{\sqrt{3}}{4}$

$(Side)^{2}$

$=\frac{\sqrt{3}}{4} \times(28)^{2}$

$=196 \sqrt{3}$

$=333.2 \mathrm{~cm}^{2}$
Area of sector

$\mathrm{OAPB}=\frac{60}{360} \times \pi r^{2}$

$=\frac{1}{6} \times \frac{22}{7} \times 28 \times 28$

$=\frac{1232}{3} \mathrm{~cm}^{2}$
Now,
Area of segment

$\mathrm{APB}=$ Area of sector

$\mathrm{OAPB}$ - Area of

$\triangle \mathrm{OAB}$

$=\left(\frac{1232}{3}-333.2\right) \mathrm{cm}^{2}$
Area of design

$=6 \times\left(\frac{1232}{3}-333.2\right)$

$=2464-1999.2$

$=464.8 \mathrm{~cm}^{2}$
Cost of making

$1 \mathrm{~cm}^{2}$ designs

$=$ Rs 0.35
Cost of making

$464.76 \mathrm{~cm}^{2}$ designs

$=464.8 \times 0.35$

$=\operatorname{Rs} 162.68$
Hence, the cost of making such designs would be Rs

$\mathbf{1 6 2 . 6 8}$ .

14. Tick the correct answer in the following:
Area of a sector of angle p (in degrees) of a circle with radius R is
A.

$\frac{P}{180} \times 2 \pi R$ B.

$\frac{P}{180} \times \pi R^{2}$ C.

$\frac{P}{380} \times 2 \pi R$ D.

$\frac{P}{720} \times 2 \pi R^{2}$

Answer

Area of sector of angle

$\theta=\frac{\theta}{360} \times \pi R^{2}$
Where

$\theta=$ angle,

$r=$ radius of circle
Here

$\theta=\mathrm{p}$ and radius

$=\mathrm{R}$
Area of sector of angle

$\mathrm{p}=\frac{p}{360} \times\left(\pi R^{2}\right)$
Multiply and divide by 2,
Area of sector of angle

$\mathrm{p}=\left(\frac{p}{360}\right)\left(2 \pi \mathrm{R}^{2}\right)$
Hence, (D) is the correct answer

NCERT Solutions for Class 10 Maths Chapter 12: Areas Related to Circles || CBSE Class 10 Maths Chapter 12 Constructions solutions Ex 12.2

Download the Math Ninja App Now